Legume Genomics and Genetics 2014, Vol.6, No.1, 1-11
6
blocks. Plots consisted of two rows each of length 1 m.
The distance between rows was 30 cm and between
plants along the row was 10 cm. To avoid any border
effect, plots were surrounded by a row of
non-experimental material. The observations were
recorded on ten quantitative traits
viz.
days to 50%
flowering, days to maturity, plant height, number of
primary branches/plant, number of cluster/plant,
number of pods/plant, number of seeds/plant, pod
length, 100 seed weight and yield/plant. Analysis of
data for general and specific combining ability was
carried out following Griffing’s (1956) Method II,
Model I (fixed effect model). The statistical analysis
was carried out using (AGRISTAT) software.
Table 1 Varieties/Landraces used in hybridization programme
Serial No. Varieties/Landraces used in
hybridization programme
Remark
1
B-3-8-8
Released variety
2
OBG-17
Released variety
3
TU-94-2
Released variety
4
PU-30
Released variety
5
PU-35
Released variety
6
LBG-17
Released variety
7
OBG-31
Released variety
8
Keonjhar Local
Local landrace
The combining ability analysis has been worked out
according to the procedure suggested by Griffing’s
(1956) Method II, Model I. The mathematical model
for the combining ability analysis is assumed to be:
Y
ijkl
= μ + g
i
+ g
j
+ s
ij
+ 1/bcΣi Σeijkl
(ij = 1, 2, 3 ….n;k = 1, 2, 3,………b;l = 1, 2, 3 ….c)
Where Y
ijkl,
Mean of i × jth genotype in k
th
replication;
μ, the population mean; gi, the GCA effect of i
th
parent;
gj, the GCA effect of j
th
parent; s
ij
, the specific
combining ability (sca) effect for the cross between i
th,
j
th
parent such that s
ij
= s
ji
; Σi Σeijkl, the
environmental effect associated with the ijkl
th
individual observation on i
th
individual in the k
th
block
with i
th
as female parent and j
th
as male parent. The
usual restrictions such as Σgi = 0 and Σsij = sii = 0 (for
each i) were imposed.
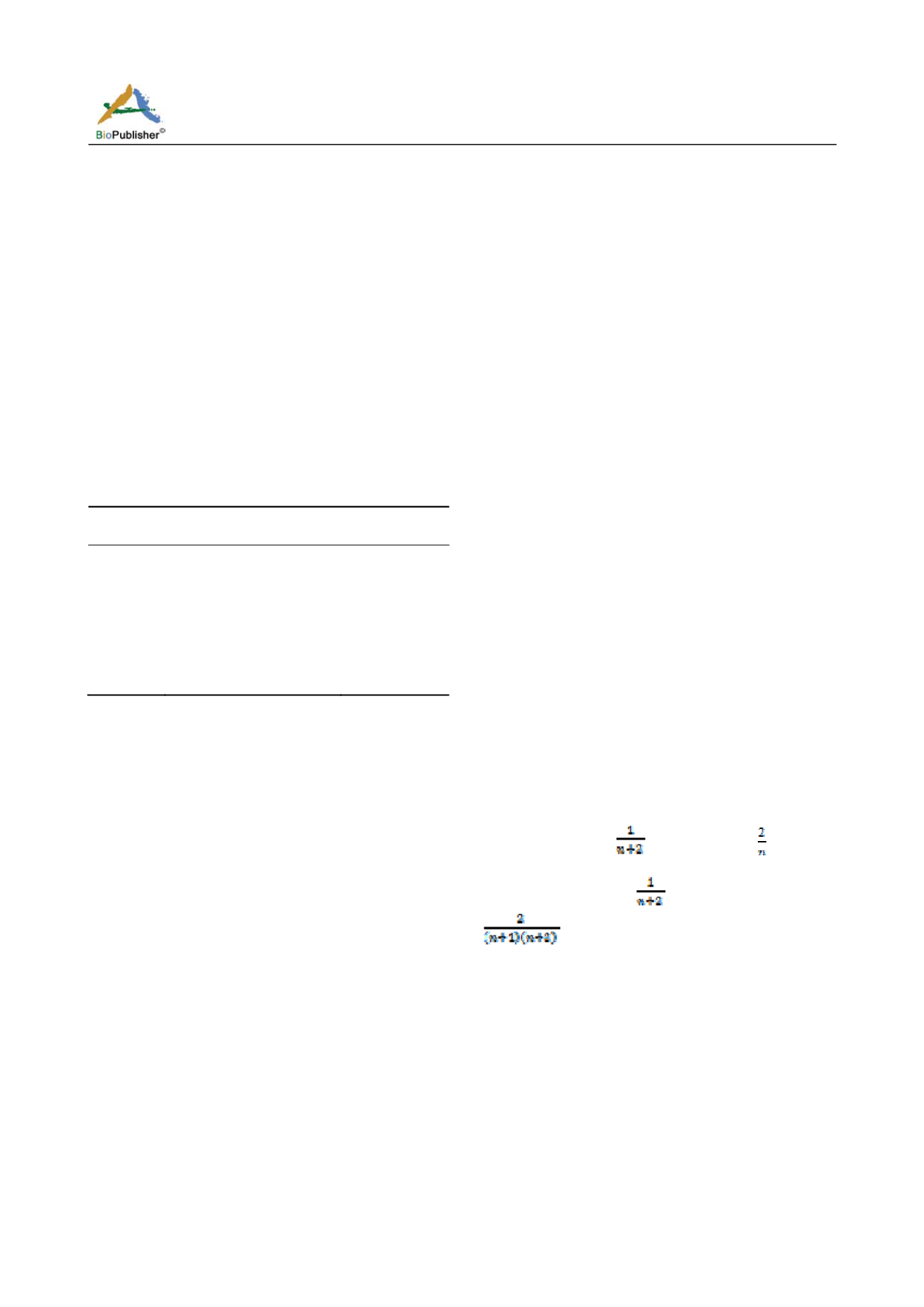
[Σ (Yi + Yii)
2
– (4/n) Y
2
], Ss = Sum of squares due
to sca,
Ss=Σ < Σ Y
2
ij – 1/ (n + 2) [Σ (Yi + Yii)
2
+ 2/ (n + 1)
(n + 2) Y
2
],
Me’ =Me1/r
Where Y
i
, Total of the array involving i
th
as a female
parent; Y
ii
, the value of the i
th
of the array; Y.., the
grand total; Y
ij
, the value of i x j
th
cross; MeI, the error
M.S (Mean square) obtained from main ANOVA. The
components of variances were estimated as suggested
by Singh
et al.
(2003) in the following ways:
GCA expected M.S. =σ
2
e
+ (n + 2)/ (n – 2) σ
2
gi
SCA expected M.S. = σ
2
e
+ 2/(n – 1)
2
sij
Estimates of various effects
The various effects were estimated as follows:
GCA effect of i
th
parent = g
i
= 1/(n+2) [(Yi. + Yii) –
2/nY..]
SCA effect of ij
th
cross = S
ij
= Yij – 1/ (n + 2) [Yi. +
Y.j + Yij) + 2Y/ (n + 1) (n +2)Y..]
Where gi and sij, The estimates of the GCA and SCA
effects, respectively; n, Yi, Yii, Y.. and Y
ij
, the same as
explained earlier; Y.j, total of the arrays involving jth
parent as a male; Y
jj
, the value of the jth parent in the
array.
Estimation of combining ability effects
The general combining ability (GCA) effects and
specific combining ability (SCA) effects were
estimated as follows.
GCA effects = g
i
= [∑ (X
i
+ X
ij
) - X......]
SCA effects = S
ij
= X
ij
(X
i
+ X
ij
+ X
j
+ X
ij
) +
X......
Where,
X
i
= total of j
th
column
X
ij
= value of j
th
parent
Authors’ Contributions
KKP carried out the overall experiment, AM and JP prepared
the manuscript. BB and MK supervised the experiment as
Chairman and member of the advisory committee for the
doctoral degree thesis work.
Reference
Bhagirath R., Tikka S.B.S., and Acharya, S., 2013, Heterosis and combining
ability in blackgram (
Vigna mungo
) under different environments,


